• Trigonometric Functions
  Sine, cosine, tangent, inverse — the easy way.
• Hyperbolic Functions
  How to enter them with one finger.
• Reciprocal Functions
  Using cosecant, secant, cotangent etc.
• Easier Radians
  Making radians more understandable.
• Useful Tips
  
  • Working with degrees and radians
  • Entering degrees, minutes, seconds
  • The ‘Double Key’ technique
  • Embrace the unknown

Let’s calculate the height of a triangle.

From the definition of sine, the height is

8 × sin 30°

Or more concisely 
8 sin 30°

So you enter:

8 sin 30

By default, angles are in degrees, hence 30 is displayed as 30°.

The Angle button is both a switch and an indicator.

DEG

It switches between degrees and radians. When it indicates DEG,
your angles are in degrees; otherwise RAD for radians.

---

The shortcuts are quite easy to remember.

sin	S
cos	C
tan	T

For 8 sin 30, you can type:

8  S  30

---

Inverse

Sometimes we need to find the angle. For example:

We know that

Therefore the angle is the inverse sine or the arcsin of 0.5.
We write it as  sin^–1 0.5.

---

Entering sin^–1 — The traditional way

Mouse:	Click	⇧	follow by	sin
Keyboard:	Hold	shift	and press	S  at the same time

This method is common amongst traditional calculators.
However it’s a bit awkward to press two keys at once.

---

Entering sin^–1 — The natural way

Let’s look at it visually.

sin^–1 has 2 parts.

It turns out there are buttons for them.

sin	sin
inverse	x–1

All you need is to enter sin and ^–1.

The shortcut for inverse is the single quote ( ' ).
Yes you can think visually too.

How to enter

We write hyperbolic sine as:

Again think of it as 2 parts:

Therefore you enter sin, then h.

---

The inverse is similar.

These are its parts:

This is how you enter:

These are cosecant (csc), secant (sec), cotangent (cot).
There are 2 ways to enter.

---

The easy way — via mouse

Click and hold the button sin, cos, or tan:

---

The efficient way — via keyboard

The is quite interesting. More rewarding too.

First, let’s meet our new friend, inverse transformation.
It’s under the menu Calculation ▸ Inverse.  Shortcut: ⌘ '

You can use it to invert a number.
E.g.  enter 4 and press ⌘ ' for 1/4. You get 0.25.

Now let’s get back to csc, sec, cot.
Knowing that they are reciprocals of sin, cos, and tan…

And inverse transformation can give us the reciprocal…

Yes you can invert trigonometric functions:

So to enter csc, press:

sin  ⌘ '

Since the shortcut for sin is S, you can simply enter:

S  ⌘ '

Working in degrees is easier because we are dealing with simple numbers. For example:

In contrast with radians, we face with messy numbers.

In degrees, 360° is one revolution, so it’s easy to see 90° is ¼ revolution.
In radians, it’s hard to see 1.570796 is one half of π.

---

But we can view the result as a multiple of π.
Go to:  Menu ▸ Angle ▸ Unit Options ▸ × π

If you want you can see it as a fraction by pressing ⌘/.

Tip

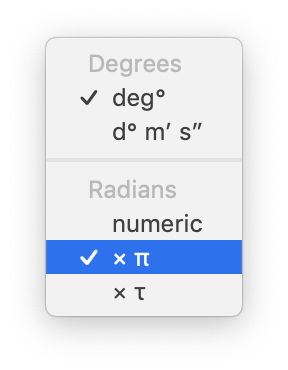
You can access the radians option quicker.
Just click and hold the RAD button to see its menu.

---

Introducing tau, τ

While ½ π is more approachable than 1.570796, still we have to do the extra arithmetic to see this angle naturally as ¼ revolution.

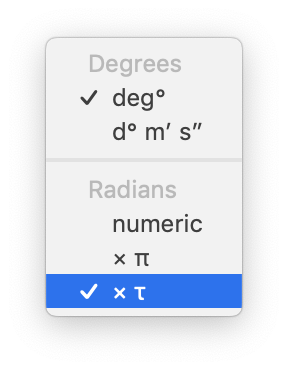
This is where tau can help because
τ  =  2 π

That is,
1 τ  radians  =  1 revolution

This makes radians more intuitive, more so than degrees:

We can go on. But we will let these smart people do the talking.

---

To view radians as a multiple of τ, click and hold the RAD button

And in fraction form:

---

Entering angles in radians

45° is 0.785398 radians. In practice it’s quicker and more accurate to type it as π / 4 or τ / 8.

Constant	Name	Shortcut
π	Pi	P
τ	Tau	⌥T

Generally one has to put parentheses around them, for instance, “tan (π / 4)”.

As π and τ are angular constants, if you use them for trigonometry, parentheses are not required.

The simple way:

Working with degrees and radians

You can switch between degrees and radians via the Angle button.

DEG  ⟷  RAD

Shortcut: ⌥⌘R.

If you want to convert one unit to another, go to menu
Calculation ▸ Extra Functions ▸ Trigonometry (⇧⌘F).

---

Entering degrees, minutes, seconds

Use the time format ‘hour : minute : second’.
It is analogous to degree° minute′ second″

For sin 30°40′50″, enter sin 30:40:50.

---

The ‘Double Key’ technique

Buttons like sin has a secondary function, sin^–1, you access it by pressing its shortcut while holding shift (⇧). It’s a two-finger hassle.

⇧+S → sin^–1

With Double Key, you can do it with one finger — just hit S twice.

SS → sin^–1

Likewise for cos^–1 and tan^–1.

CC → cos^–1

TT → tan^–1

And for the adjacent function you learned earlier:

HH → adjacent

---

Embrace the unknown

We spent a lot of time talking about the inverse. The truth is, you don’t have to use it.

Earlier we calculated an angle with sin^–1 0.5, you get achieve the same by entering sin ? = 0.5.

? is the unknown, just like ‘x’ in algebra.

Here is an example with the law of sines:

Click here to learn more about the unknown.